Visibility of Highway Signs

Why did the researchers randomize whether a participant saw the text signs or the symbolic signs first?

 

• Understand the notion of confounding. Understand how randomization of group assignments yields comparison groups that are similar with respect to confounding factors.

They randomized the order so that if there happened to be some sort of "learning effect" (i.e. recognizing the second group of signs at a smaller size because you had an idea after the first group what to expect) it would affect both groups and not favor one over the other.

Graphically explore the relationships among the threshold sizes of the three versions of the signs. What do you find?

 

• Be able to use your software to make a scatterplot. Be able to interpret the display (for example, recognizing patterns or spotting outliers).
• Be able to make (using software where appropriate) and interpret the principle methods of displaying distributions (frequency tables, pie charts, line graphs, bar graphs, and histograms).

One option is to make boxplots to compare the threshold sizes of the text signs to the symbolic signs, putting both types of symbolic signs together.

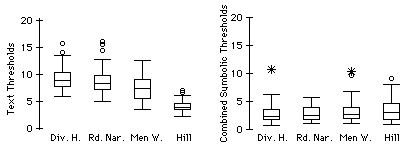
Except for the Hill sign, it appears that the symbolic signs were visible at a much smaller size than the text signs.

One could also compare the two types of symbolic signs to each other.

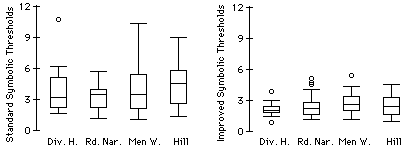
Though the difference between the centers of the two groups is not as striking as between the text and symbolic versions, it does appear that the threshold sizes of the improved signs are smaller than the threshold sizes for the standard symbolic ones. We can also see that there is less variation among the improved thresholds than the standard thresholds.

We are interested in exploring whether the improved symbolic signs are more visible than their standard counterparts.

a) Estimate the mean difference between standard and improved threshold sizes for each of the four types of signs. Write a sentence interpreting what one of these estimates tells us.

b) Kline and Fuchs (1993) used a significance level of 0.01 for all of their inferences. Why do you think they used the more conservative value of 0.01 rather than the common 0.05?

c) Construct a 99% lower confidence bound for the mean difference between standard and improved threshold sizes for each of the four signs. Interpret your results.

d) Why did we construct a one-sided confidence bound in Part c rather than a two-sided confidence interval?

• Be able to make confidence intervals for proportions and means from a simple random sample.
• Understand that the construction of a confidence interval for the mean depends on whether or not we know the population variance, σ. Be able to use the appropriate distribution when computing the margin of error in either case (i.e. when σ is known AND when it is not known).
• Be able to interpret confidence intervals in lay terms.
• Understand that the P-value is the probability of getting a sample statistic as extreme or more extreme than what was seen in the sample given that the null hypothesis is true.
• Understand that P-values are strongly related to sample size. Be aware that statistical significance and practical significance are different.

a) If we let  be the observed average standard threshold size and  be the observed average improved threshold size, then our estimate of the mean difference is - .. For the four signs the estimates are:

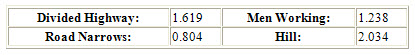
We can say, "On average, the threshold size of the improved Divided Highway sign is 1.619 cm smaller than that of the standard Divided Highway sign."

b) Since it would be very costly to change highway signs if the improved versions are deemed better, the researchers wanted to guard against inappropriately suggesting a change in the current system. They wanted to make sure they had strong evidence before suggesting any possible changes. Therefore they used a significance level of 0.01.

c) We can use the formula ( − ) − t where = which yields a lower confidence bound for two-sample data, where t is the appropriate cut-off from the t distribution. We can calculate the degrees of freedom for t in two ways, either with Welch's approximation or with min(n_x − 1, n_y − 1) = 23. (The second method will yield smaller bounds than the first.)  The resulting bounds are:

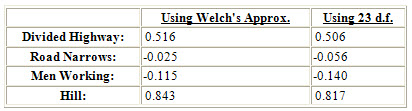
Notice that the bounds for the Road Narrows and Men Working signs are negative. At the 99% confidence level we do not detect a significant difference in threshold sizes for these two signs. For the Divided Highway and Hill signs, however, we are 99% confident that the threshold sizes for the improved symbolic signs are at least 0.516 and 0.843 cm smaller than those for the standard versions respectively.

d) We use a one-sided lower bound because we are only interested in detecting whether the improved signs are significantly better than the standard ones. If the improved and standard signs are equally good or if the data showed the standard signs are better than the improved versions, we would continue to use the standard signs. Only if the improved signs are considered significantly better would we change what we are doing now.

To help us evaluate what practical significance there is in the difference in threshold sizes of, say, a half of a centimeter, we can convert the threshold sizes into another set of units called sight time. Sight time is the time in seconds that a person traveling at 60 mph would have after recognizing a sign until he or she reached the sign. To calculate sight time we use the formula sight time = constant/threshold size. The constant is calculated from the size of the actual road sign, the distance the subject sat from the monitor during the experiment, and the assumed 60 mph speed. The following table gives the average sight times for the three types of signs and the three age groups.

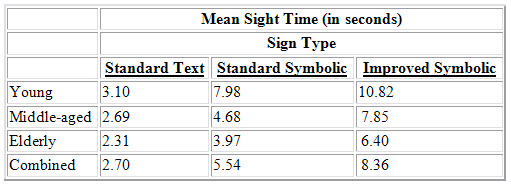
In each age category, people on average would be able to recognize improved symbolic signs about three seconds faster than the standard symbolic counterparts. Do you think that three seconds is enough of a practical significance to justify changing the current road signs? What course of action would you recommend to the highway administration?

 

• Understand that the P-value is the probability of getting a sample statistic as extreme or more extreme than what was seen in the sample given that the null hypothesis is true.
• Understand that P-values are strongly related to sample size. Be aware that statistical significance and practical significance are different.

The practical significance of three seconds is debatable. On one hand, having eight seconds instead of five to respond may be enough of an improvement to prevent possible accidents. On the other hand, the cost of replacing existing road signs would be prohibitive, and you might argue that five seconds is more than enough time, especially considering text signs only afford three seconds of reaction time. Perhaps you might wish to recommend slowly phasing the new designs into production, and using the improved signs when new signs are needed. It may also be helpful to know the sight times for other speeds such as 50 mph or 35 mph. Information on the average amount of time a driver needs to properly react would be helpful in deciding if three seconds is worth the cost.

a) Consider now the threshold sizes of the text version of the Road Narrows sign. Graphically explore the relationship between a participant's threshold and his or her age.

b) What do you think is causing most of the apparent association between these two variables? If possible, find a variable in the data set that could be a possible confounding factor between threshold and age. One way to do this is to plot possible variables against both threshold and age and see if any are correlated with both. What do you find?

c) Make a scatterplot of the Road Narrows text threshold versus best eye acuity. (Note: a higher acuity measurement indicates poorer vision.) What is the correlation?

d) Instead of the threshold size, suppose we are interested in the size of the letters on the sign when the threshold measurement was taken. Do you think the correlation between the letter size and best eye acuity will be greater than, less than, or equal to the correlation between threshold size and best eye acuity? Why? Verify your guess by computing the correlation.

• Be able to use your software to make a scatterplot. Be able to interpret the display (for example, recognizing patterns or spotting outliers).
• Be able to use software to calculate the correlation coefficient. Be able to interpret this value.

 

a) There seems to be a moderate association between threshold size and age, with higher thresholds corresponding to older subjects.

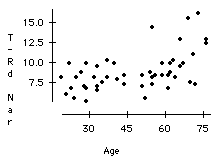
It does appear, however, that the association is not completely linear. The relationship is relatively flat until about age fifty, where there starts to be an increase.

b) When we plot acuity versus both the text threshold and age, we see that both plots show a positive correlation.

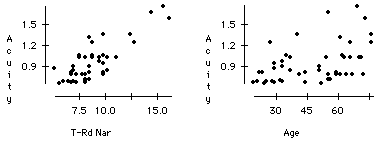
As people age, their eyesight tends to worsen, and people with poor eyesight require larger thresholds to recognize the sign. Thus it does appear that acuity may be a confounding factor between threshold size and age.

c)

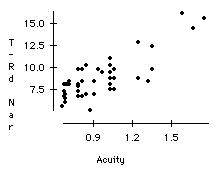
The correlation is 0.810. Higher thresholds correspond to higher acuity levels.

 

d) We would expect the correlation to be the same. The ratio of letter height to sign height is constant for a particular sign. For any particular subject, if we divide the threshold size of the Road Narrows sign by the letter height threshold we get approximately 8.86. (There is some error due to rounding and measurement error.) Multiplying one of the variables by a constant does not affect the correlation between two variables. The correlation is again 0.810.

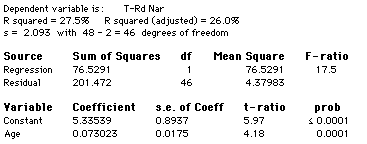
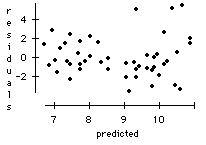
a) Regress the thresholds of the text Road Narrows sign on age. Look at the residual plot. Do you see anything unusual?

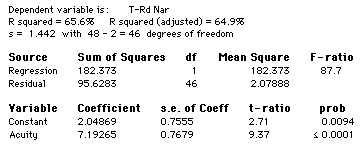
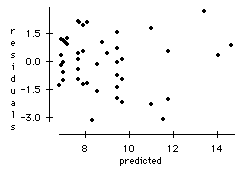
b) Try adding acuity to the regression model. Does this variable add anything to the model? Look at a plot of residuals. Do you see anything unusual? Which model do you prefer, the one using age or acuity? Why?

• Be able to use the computer to calculate simple linear regression estimates and know how to interpret the resulting output including (y = response variable,= estimated response, and x = the explanatory variable): the intercept (the value ofwhen x = 0), the slope (the amount by whichchanges when you increase x by one unit), the correlation (the measure of the strength and direction of the straight line relationship between x and y), the R-squared value (the square of the correlation measures the proportion of the variance of one variable that can be explained by straight-line dependence on the other variable), and RMSE (the new standard deviation of y after you know x).
• Understand residuals

 

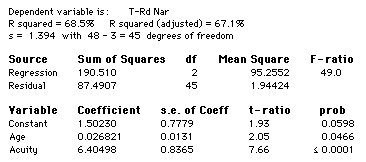
a) The regression output below indicates that age is a significant predictor in this model, but the value of R² = 27.5% is fairly low. The residual plot below shows no striking pattern.

b) Basic version
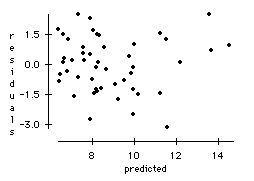
This model looks better than the one using age. The value of R² = 65.6% is much higher. In other words, 65.6% of variability in T-Rd Nar is explained by the regression on Acuity. The residual plot below for this model shows no obvious problems.

Semi-Tech version
Adding acuity improves the model quite a bit. The value of R² has increased to 68.5% and the residual plot below shows no obvious problems.